Transpositional Invariance

The TransformSynth presents several examples of tuning continua - parameterized families of tunings where each specific tuning corresponds to a particular value of the parameter. These tuning continua are transpositionally invariant as described here (where each interval, chord, and scale type has the same geometric shape in every key) as well as tuning invariant (where all intervals and chords within a specified set have the same geometric shape in all of the tunings of the continuum). For example, in the syntonic continuum, a major chord can be played like this on a hexagonal keyboard.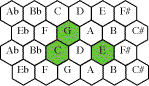




This pattern always plays a major chord. Shifted one to the left, it descends by a whole step. Below and the left lies the fourth. Below and to the right is the fifth. Here are several different major chords. All have the same geometrical shape.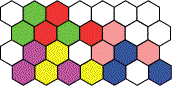




This is an example of transpositional invariance. Anywhere this pattern appears the same chord. Similarly, the minor chord has the same shape in all keys. Minor chords look like this: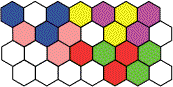




Doing this requires a two dimensional keyboard surface (instead of a linear, one dimensional keyboard as is used on a piano). For learning about these, you can use the keyboard of your computer. Hopefully soon there will be musically-oriented two dimensional keyboards more readily available.